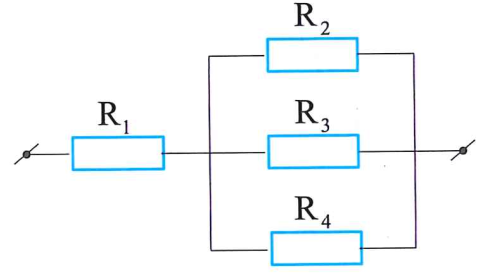
T
Bốn điện trở ${{R}_{1}}=2\Omega ;{{R}_{2}}=6\Omega...
- Tác giả The Knowledge
- Creation date