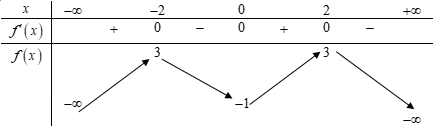
Cho hàm số $y=f\left( x \right)$ có bảng biến thiên như sau Hàm số $y=f\left( {{x}^{2}}-2 \right)$ đồng biến trên khoảng nào dưới đây?

Ta có $y'=2x.f'\left( {{x}^{2}}-2 \right)=0\Leftrightarrow \left[ \begin{aligned}
& x=0 \\
& f'\left( {{x}^{2}}-2 \right)=0 \\
\end{aligned} \right.\Leftrightarrow \left[ \begin{aligned}
& x=0 \\
& {{x}^{2}}-2=-2 \\
& {{x}^{2}}-2=2 \\
& {{x}^{2}}-2=0 \\
\end{aligned} \right.\Leftrightarrow \left[ \begin{aligned}
& x=0 \\
& x=2 \\
& x=-2 \\
& x=\sqrt{2} \\
& x=-\sqrt{2} \\
\end{aligned} \right.$
Bảng biến thiên hàm số $y=f\left( {{x}^{2}}-2 \right).$
Từ bảng biến thiên ta thấy hàm số đồng biến trên khoảng $\left( -\infty ;-2 \right).$